Problem
A Metal Rod with length 1.2 m and mass 500 g is suspended in a magnetic field of 0.9 T. Determine the current needed suspend the rod without supports.
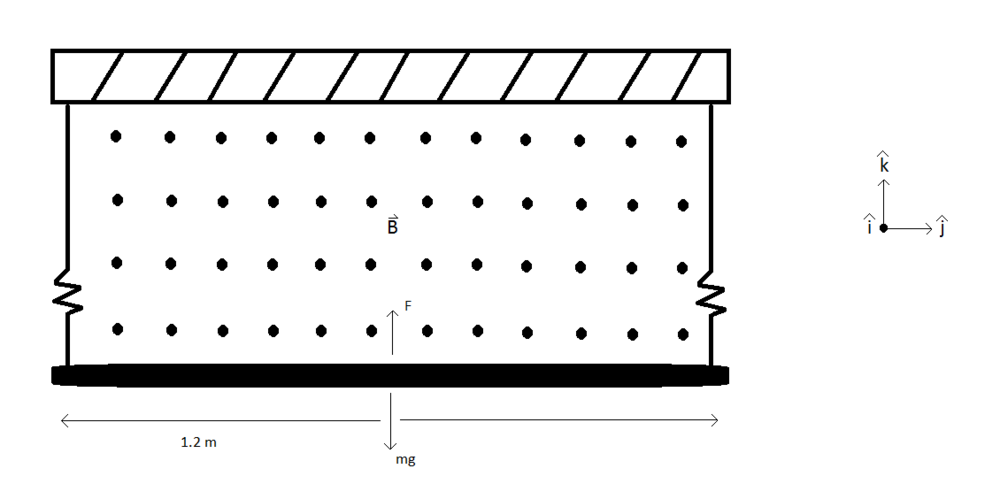
Solution
Ampere's Force Law

For our problem we have 
And we know the necessary Force to hold up the bar without the supports would be equal to the weight of the bar multiplied by the gravitational force.
We also know that the force vector will be in the  direction, and B is in the
direction, and B is in the  direction.
direction.
So using the fact that  We can conclude that the current is in the
We can conclude that the current is in the  direction, and therefore flows to the left.
direction, and therefore flows to the left.



Substituting into Ampere's Law we are left with

Integrating from  gives us
gives us

In conclusion we would need 4.54 Amps of current flowing to the left to suspend the bar in the magnetic field.

Review Comments and Status
Andrew Sell- emailed 1.25.10
Kyle Lafferty- emailed 1.25.10













