Nick ENGR431 P1

Nick Christman: Paper 1 - Magnetostatics

Back to Nick's EMEC Wiki

Back to Class EMEC Wiki

Brief Introduction to Magnetostatics

Nick Christman

According to several sources, there is an ancient story about a Cretan shepherd named Magnes who accidentally discovered a naturally occurring magnetic material known as lodestone (or loadstone). As the legend goes, Magnes was herding his sheep one day when the iron nails in his shoes and the iron tip on his staff became unusually attracted to a large, dark stone – lodestones contain a mineral, now known as magnetite ($Fe_{3}O_{4}$), that consists of naturally occurring magnetic properties<ref>Jezek, How Magnets Work</ref>. The first record of using magnets is somewhat of a debatable topic because magnetic properties were first recorded by Greek philosophers possibly as early as the 7th century BC and the Chinese have written records dating circa 4th century BC. In either case, the awareness of magnetic properties has been around for quite some time and the study of magnetism continues to be important and popular portion of research topics.

Magnetostatics, the study of static magnetic fields, is only a small portion of the overall study of magnetic properties. As just stated, magnetostatics implies that the magnetic field is static – that is, the flow of current that creates the magnetic field is steady or direct current (DC)<ref>Wikipedia, Magnetostatics</ref> – and this allows scientists to make very accurate approximations of how magnetic fields act. In this document, the theory and application behind magnetostatics will be addressed.

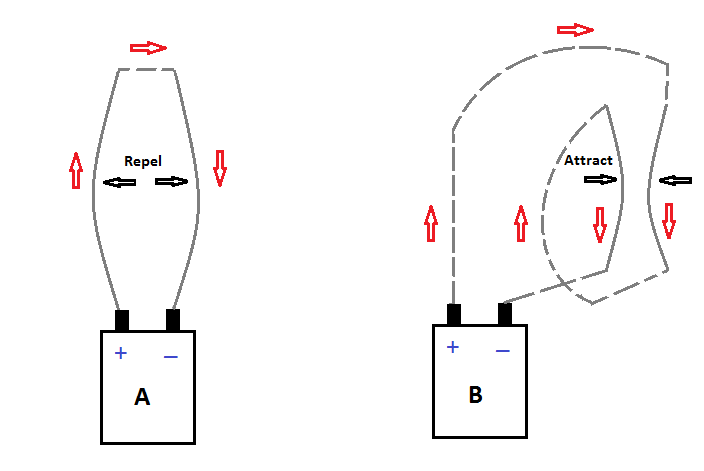
It is said that currents in opposite directions repel while currents in the same directions attract. To demonstrate this, take a common example that is illustrated in David Griffiths Introduction to Electrodynamics, 3rd edition: “[Imagine] two wires hang from the ceiling, a few centimeters apart; when I turn on a current so that it passes up one wire and back down the other, the wires jump apart. Moreover, I could hook up my demonstration so as to make the current flow up both wires; in this case they are found to attract” <ref>Griffiths, p. 203</ref>(Griffiths 203). This demonstration is illustrated in Figure 1. Through experimentation it has been proven that the forces of attraction in this system are not due to electrostatics, but instead are the result of magnetic forces – in addition to an electric field, a moving charge also generates a magnetic field<ref>Griffiths, p. 203</ref> (Griffiths 203). In fact, if one were to allow a current to flow down a straight wire, then a magnetic field circling the wire will be formed – recall the right-hand-rule which states that if you grasp the wire with your right hand (thumb pointing in the direction of current) then your fingers curl in the direction of the magnetic field. Place two wires in close proximity to each other, such as those mentioned in the demonstration above, and there will be a magnetic force between the two wires – hence, in one case the two wires repel and in the other they attract.

Figure 1: Illustration of (A) currents in opposite directions repel and (B) currents in the same directions attract. Image created by Nick Christman.

This concept of magnetic force is an interesting, yet complicating topic that is often covered in series of undergraduate and graduate level courses. Generally speaking, however, there is one law that encapsulates all of magnetostatics, which is Lorentz Force Law

$\textstyle F=Q(v\times B)$<ref>Griffiths, p. 204</ref>

where Q is the charge for which the magnetic field is acting upon, B is the magnetic field, and v is the velocity of the charge Q. (Note that this force, F, is due to the magnetic field acting on Q and that there are no external electric fields present. In the presence of an external electric field, there would be an additional term.) Furthermore, Lorentz Force Law can be expanded in order to account for a current carrying wire rather than a point charge. In the case of a current carrying wire, Lorentz Force Law becomes

$\textstyle F=\int (I\times B)dl=\int I(dl\times B)$<ref>Griffiths, p. 204</ref>

where $I$, the current, is represented by a vector pointing in the same direction as $dl$. In most cases, however, current is treated as constant; thus, Lorentz Force Law can be written as

$\textstyle F=I\int (dl\times B)$<ref>Griffiths, p. 209</ref>

Overall, magnetostatics has been derived from a compilation of different sources and it is used to more simply model the properties of magnetism. In both the constant and non-constant current cases presented above, it is clear that the Lorentz Force Law is an important aspect of magnetostatics that can be expanded to account for nearly every study of magnetism. This short overview of magnetostatics is important when one desires to develop and understand magnetic circuits and, ultimately, transformers.

---

References:

Griffiths, David J. "Introduction to Electrodynamics." Upper Saddle River, N.J: Prentice Hall, 1999.

Jezek, Geno. "History of Magnets." How Magnets Work. Web. 9 Jan. 2010. <http://www.howmagnetswork.com/history.html>.

Mohan, Ned. Electric Drives An Integrative Approach. Minneapolis: Mnpere, 2004.

Wikepdia. "Magnetostatics." Wikipedia, the free encyclopedia. Web. 10 Jan. 2010. <http://en.wikipedia.org/wiki/Magnetostatics>.

---

In-text Citations:

<references/>

---

Statistics:

Word Count: 845 (content, equations, work cited, and title)

Image Count: 1

Potential Points: TBD

---

Reviewers:

• Kevin Starkey

• Tim Rasmussen

---

Readers:

• Jimmy Apablaza
• Christopher Garrison Lau I
• Wesley Brown