Laplace Transforms: Vertical Motion of a Coupled Spring System: Difference between revisions
Jump to navigation
Jump to search
No edit summary |
|||
| Line 1: | Line 1: | ||
__TOC__ |
__TOC__ |
||
=Problem Statement= |
= Problem Statement = |
||
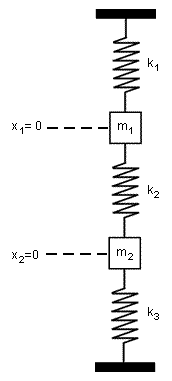
[[Image:Figure1.gif|thumb|Figure 1. Coupled Spring System.]]Derive the system of differential equations describing the straight-line vertical motion of the coupled spring shown in Figure 1. Use Laplace transform to solve the system when <math>k_1=k_2=k_3=1</math>, <math>m_1=m_2=1</math>, and <math>x_1(0)=0</math>, <math>x'1(0)=-1</math>, <math>x_2(0)=0</math>, and <math>x'_2(0)=1</math>. |
[[Image:Figure1.gif|thumb|Figure 1. Coupled Spring System.]]Derive the system of differential equations describing the straight-line vertical motion of the coupled spring shown in Figure 1. Use Laplace transform to solve the system when <math>k_1=k_2=k_3=1</math>, <math>m_1=m_2=1</math>, and <math>x_1(0)=0</math>, <math>x'1(0)=-1</math>, <math>x_2(0)=0</math>, and <math>x'_2(0)=1</math>. |
||
= Solution = |
|||
At positions <math>x_1</math> and <math>x_2</math>, the masses <math>m_1</math> and <math>m_2</math> are in equilibrium. Thus, the motion equations for <math>m_1</math> and <math>m_2</math> are, |
|||
<math>m_1x''_1=-k_1x_1+k_2(x_2-x_1)</math> |
|||
<math>m_1x''_1+k_1x_1-k_2(x_2-x_1)=0</math> |
|||
<math>m_2x''_2=-k_2(x_2-x_1)-k_3x_2</math> |
|||
<math>m_2x''_2+k_2(x_2-x_1)-k_3x_2=0</math> |
|||
where <math>m_1x''_1</math> and <math>m_2x''_2</math> represent the [http://en.wikipedia.org/wiki/Newton%27s_laws_of_motion Newton's Second Law of Motion] and <math>-k_1x_1+k_2(x_2-x_1)</math> and <math>-k_2(x_2-x_1)-k_3x_2</math> represent the net forces acting in the masses. |
|||
== Laplace Transform == |
|||
Applying the Laplace Transform to the motion equations for this systems, we obtain, |
|||
<math>L[m_1x''_1]=L[-k_1x_1+k_2(x_2-x_1)]</math> |
|||
<math>L[m_2x''_2]=L[-k_2(x_2-x_1)-k_3x_2]</math> |
|||
Revision as of 11:40, 2 November 2009
Problem Statement
Derive the system of differential equations describing the straight-line vertical motion of the coupled spring shown in Figure 1. Use Laplace transform to solve the system when , , and , , , and .
Solution
At positions and , the masses and are in equilibrium. Thus, the motion equations for and are,
where and represent the Newton's Second Law of Motion and and represent the net forces acting in the masses.
Laplace Transform
Applying the Laplace Transform to the motion equations for this systems, we obtain,


















![{\displaystyle L[m_{1}x''_{1}]=L[-k_{1}x_{1}+k_{2}(x_{2}-x_{1})]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/6902b2a4e082ac83009328a754c1167b987c605e)
![{\displaystyle L[m_{2}x''_{2}]=L[-k_{2}(x_{2}-x_{1})-k_{3}x_{2}]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/60954d59881330be1873accb1bb05ee37df8b854)