Magnetic Circuit: Difference between revisions
John.hawkins (talk | contribs) (Starting) |
|||
| (14 intermediate revisions by 2 users not shown) | |||
| Line 1: | Line 1: | ||
=Author: John Hawkins= |
|||
==Problem Statement== |
==Problem Statement== |
||
| Line 6: | Line 7: | ||
==Solution== |
==Solution== |
||
First, we note that the problem statement is incomplete. |
First, we note that the problem statement is incomplete. Assume that the core has a relative permeability of 500. Hence, for all magnetic sections excluding the air gap, |
||
<center><math>\mu=\mu_r\mu_0=(500)(4\pi\times10^{-7})=6.2832\times10^{-4}</math></center> |
|||
| ⚫ | |||
Also, as recommended in the text, we will neglect fringing. |
|||
The lengths and areas of each of the sections to be evaluated are given in the following table. |
|||
{| class="wikitable" border="1" |
|||
|+ ''Table 1: Lengths and Areas for the pertinent secions of the magnetic circuit.'' |
|||
! '''Section''' !! fg !! def !! ghc !! dc !! dabc |
|||
|- |
|||
| Length <math>l</math> (m)|| 0.01 || 0.555|| 0.555 || 0.48 || 1.38 |
|||
|- |
|||
| Area <math>A</math> (m<sup>2</sup>) || 0.0032 || 0.0032 || 0.0032 || 0.0096 || 8.0e-4 |
|||
|} |
|||
| ⚫ | |||
<br /> |
|||
'''Air Gap:''' |
|||
<br /> |
|||
<center> |
|||
<math>\mathcal{R}_{fg}=\frac{l_{fg}}{\mu A_{fg}} = 4973.6</math> |
|||
<br /> |
|||
<math>\Phi_{fg}=B_{fg}A_{fg}=3.20\times10^{-4}</math> |
|||
<br /> |
|||
<math>\mathcal{F}_{fg}=\mathcal{R}_{fg}\Phi_{fg}=1.5915</math> |
|||
</center> |
|||
<br /> |
|||
<br /> |
|||
'''Right Arms:''' |
|||
<br /> |
|||
<center> |
|||
<math>\Phi_{def}=\Phi_{ghc} = \Phi_{fg} = 3.20\times10^{-4}</math> |
|||
<br /> |
|||
<math>\mathcal{R}_{def}=\mathcal{R}_{ghc}=\frac{l_{def}}{\mu A_{def}}=2.7603\times10^5</math> |
|||
<br /> |
|||
<math>\mathcal{F}_{def}=\mathcal{F}_{ghc}=\mathcal{R}_{def}\Phi_{def}=88.331</math> |
|||
</center> |
|||
<br /> |
|||
<br /> |
|||
'''Center Column:''' |
|||
<br /> |
|||
<center> |
|||
<math>\mathcal{F}_{dc}=\mathcal{F}_{def}+\mathcal{F}_{fg}+\mathcal{F}_{ghc}=178.25</math> |
|||
<br /> |
|||
<math>\mathcal{R}_{dc}=\frac{l_{dc}}{\mu A_{dc}}=79,577</math> |
|||
<br /> |
|||
<math>\Phi_{dc}=\frac{\mathcal{F}_{dc}}{\mathcal{R}_{dc}}=0.0022</math> |
|||
</center> |
|||
<br /> |
|||
<br /> |
|||
'''Left Arm:''' |
|||
<br /> |
|||
<center> |
|||
<math>\ \Phi_{dabc}=\Phi_{dc}-\Phi_{def}=0.0019</math> |
|||
<br /> |
|||
<math>\mathcal{R}_{dabc}=\frac{l_{dabc}}{\mu A_{dabc}}=2.745\times 10^6</math> |
|||
<br /> |
|||
<math>\mathcal{F}_{dabc}=\mathcal{F}_{dabc}\Phi_{dabc}=5,271.2</math> |
|||
</center> |
|||
<br /> |
|||
<br /> |
|||
'''Conclusions:''' |
|||
<br /> |
|||
<center> |
|||
<math>\mathcal{F}_{Total}=\mathcal{F}_{dabc}+ |
|||
\mathcal{F}_{dc}+\mathcal{F}_{def}+\mathcal{F}_{fg}+ |
|||
\mathcal{F}_{ghc}=5,627.7</math> |
|||
<br /> |
|||
<math>\mathbf{i=\frac{\mathcal{F}_{Total}}{N}=3.52 A}</math> |
|||
</center> |
|||
<br /> |
|||
Which is the quantity we were looking for. |
|||
<br /> |
|||
Calculations were performed using the following [[Magnetic Circuit Matlab Script]]. |
|||
==References== |
==References== |
||
<references /> |
<references /> |
||
==Reviewed By== |
|||
Amy Crosby |
|||
[[Kirk Betz]] |
|||
==Read By== |
|||
==Comments== |
|||
Latest revision as of 12:42, 21 January 2010
Author: John Hawkins
Problem Statement
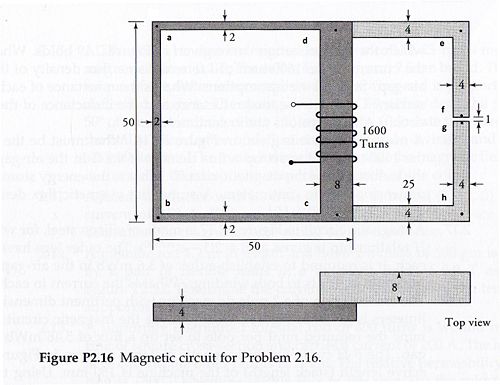
Problem 2.16 from Electric Machinery and Transformers, 3rd ed:
A magnetic circuit is given in Figure P2.16. What must be the current in the 1600-turn coil to set up a flux density of 0.1 T in the air-gap? All dimensions are in centimeters. Assume that magnetic flux density varies as .<ref>Guru and Huseyin, Electric Machinery and Transformers, 3rd ed. (New York: Oxford University Press, 2001), 129.</ref>
Solution
First, we note that the problem statement is incomplete. Assume that the core has a relative permeability of 500. Hence, for all magnetic sections excluding the air gap,
Also, as recommended in the text, we will neglect fringing.
The lengths and areas of each of the sections to be evaluated are given in the following table.
| Section | fg | def | ghc | dc | dabc |
|---|---|---|---|---|---|
| Length (m) | 0.01 | 0.555 | 0.555 | 0.48 | 1.38 |
| Area (m2) | 0.0032 | 0.0032 | 0.0032 | 0.0096 | 8.0e-4 |
We must now work backward from the air-gap, since the value of the flux-density is given there. We need only employ the analagous equations to Ohm's Law, KVL, and KCL. All units are standard units.
Air Gap:
Right Arms:
Center Column:
Left Arm:
Conclusions:
Which is the quantity we were looking for.
Calculations were performed using the following Magnetic Circuit Matlab Script.
References
<references />
Reviewed By
Amy Crosby
![{\displaystyle B=[1.5H/(750+H)]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/461efa32b8e9b84a7b09e7e5052103302f011e0f)
















