Martinez's Fourier Assignment: Difference between revisions
Jump to navigation
Jump to search
No edit summary |
No edit summary |
||
| Line 9: | Line 9: | ||
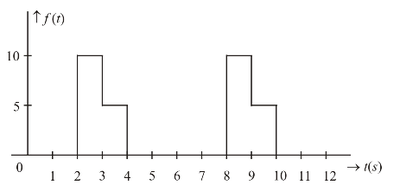
a_n &= \frac{2}{T}\int_0^T f(t)\cos(n\omega_0t)\, dt\\ |
a_n &= \frac{2}{T}\int_0^T f(t)\cos(n\omega_0t)\, dt\\ |
||
b_n &= \frac{2}{T}\int_0^T f(t)\sin(n\omega_0t)\, dt\\ |
b_n &= \frac{2}{T}\int_0^T f(t)\sin(n\omega_0t)\, dt\\ |
||
\because \!\T=6, dt\\ |
|||
\end{align} |
\end{align} |
||