Coupled Oscillator: Hellie: Difference between revisions
Jump to navigation
Jump to search
| Line 30: | Line 30: | ||
\begin{bmatrix} |
\begin{bmatrix} |
||
0&1&0&0 \\ |
0&1&0&0 \\ |
||
\frac{(k_1-k_2)}{m_1}&0&\frac{-k_1}{m_1}&0 \\ |
|||
0&0&0&0 \\ |
|||
0&0&0&1 \\ |
0&0&0&1 \\ |
||
\frac{k_1}{m_2}&0&\frac{(k_1+k_2)}{m_2}&0 |
|||
0&0&0&0 |
|||
\end{bmatrix} |
\end{bmatrix} |
||
| Line 61: | Line 61: | ||
Eigenmodes |
Eigenmodes |
||
:There are three eigenmodes for the system |
|||
::1) m1 and m2 oscillating together |
|||
::2) m1 and m2 oscillating at exactly a half period difference |
|||
::3) m1 and m2 oscillating at different times |
|||
Solve Using the Matrix Exponential |
|||
Revision as of 14:36, 25 November 2009
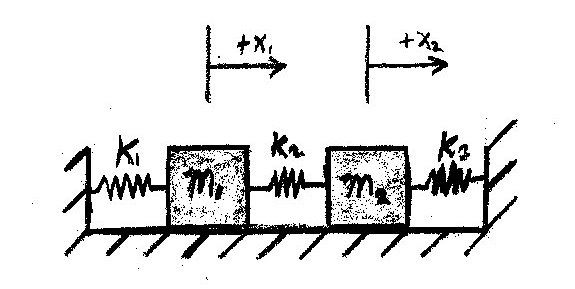
Problem Statement
Write up on the Wiki a solution of a coupled oscillator problem like the coupled pendulum. Use State Space methods. Describe the eigenmodes of the system.
Initial Conditions:
State Equations
=
Eigenmodes
- There are three eigenmodes for the system
- 1) m1 and m2 oscillating together
- 2) m1 and m2 oscillating at exactly a half period difference
- 3) m1 and m2 oscillating at different times
Solve Using the Matrix Exponential
Written by: Andrew Hellie