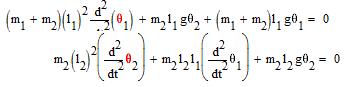Laplace transforms: Double pendulum: Difference between revisions
Jump to navigation
Jump to search
New page: '''Part 1:''' '''Problem Statement:''' Suppose two identical pendulums are coupled by means of a spring with constant K. It can be shown that when displacement angles Theta_1 (t) and the... |
No edit summary |
||
| Line 2: | Line 2: | ||
'''Problem Statement:''' | '''Problem Statement:''' | ||
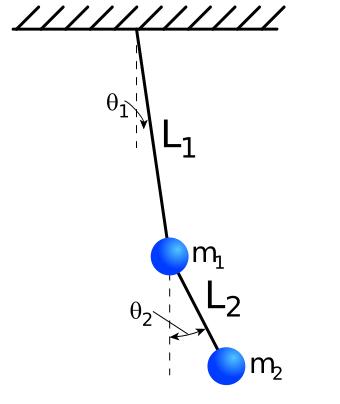
Consider the double-pendulum system consisting of a pendulum connected to a pendulum. | |||
[[Image:DP.jpeg]] | |||
If the displacements of the angles is small, the system of differential equations can be written as follows: | |||
[[Image:1.jpg]] | |||
Using Laplace Transforms | |||