Example Problem - Toroid

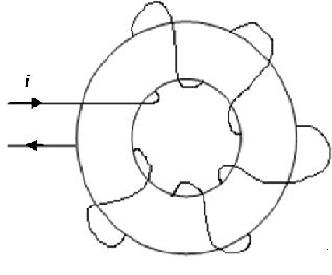
Problem: Concerning Ampere's law Let look at a coil around a toroid shown in the figure below. The coil has N = 20 turns around the toroid. The toroid has an inside diameter of ID = 4 cm and an outside diameter OD = 5 cm. Determine the field intensity H along the mean path length within the toroid with a current i = 2.5 A.

Figure created by Kirk Betz

Solution:

Do symmetry the magnetic field intensity Hm along a circular contour within the toroid is constant. We can find the mean radius by

$r_{m}=({\frac {1}{2}}){\frac {OD+ID}{2}}=2.25\ cm$

Using the mean radius the mean path of length $l_{m}$ can be calculated.

$l_{m}={2\pi r_{m}}=0.141\$

With Ampere's Law (below) the field intensity along the mean path can be Found.

$H_{m}=({\frac {Ni}{l_{m}}})$

Finally teh H_m can be calculated.

$H_{m}={\frac {20x2.5}{.141}}=354.6\ A/m)$

Since the width of the toroid is much smaller than the mean radius $r_{m}$ we can assume a uniform $H_{m}$ throughout teh cross-section of the toroid.