Basic Laplace Transforms: Difference between revisions
Jump to navigation
Jump to search
No edit summary |
No edit summary |
||
| Line 1: | Line 1: | ||
'''Basic Laplace Transforms''' |
|||
The laplace transform has the standard form of: |
The laplace transform has the standard form of: |
||
| Line 5: | Line 5: | ||
:<math>F(s) = \mathcal{L} \left\{f(t)\right\}=\int_0^{\infty} e^{-st} f(t) \,dt </math> (Cited From Fullerton, Colby) |
:<math>F(s) = \mathcal{L} \left\{f(t)\right\}=\int_0^{\infty} e^{-st} f(t) \,dt </math> (Cited From Fullerton, Colby) |
||
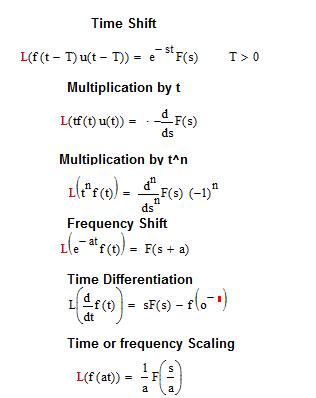
However, in this class applying the standard form exclusively to solve problems is not practical. The use of Laplace transform properties greatly simplifies problems. These properties are listed in the book on page 525. The simple properties are listed below and |
However, in this class applying the standard form exclusively to solve problems is not practical. The use of Laplace transform properties greatly simplifies problems. These properties are listed in the book on page 525. The simple properties are listed below and are imported images from mathcad. |
||
'''Linearity''' |
'''Linearity''' |
||
Revision as of 13:28, 19 January 2010
Basic Laplace Transforms
The laplace transform has the standard form of:
- (Cited From Fullerton, Colby)
However, in this class applying the standard form exclusively to solve problems is not practical. The use of Laplace transform properties greatly simplifies problems. These properties are listed in the book on page 525. The simple properties are listed below and are imported images from mathcad.
Linearity
Example:
If F(s)=(s+2)/(S+1) and f(t)=0 for t<0, then find the Laplace transform for the following functions identifying each property used to compute answers.
(a) (b)
(a) Linearity:
Time Shift: =
(b) Linearity:
Frequency Shift: