Christman GeneralizedReceiver: Difference between revisions
No edit summary |
No edit summary |
||
| Line 6: | Line 6: | ||
<br/> |
<br/> |
||
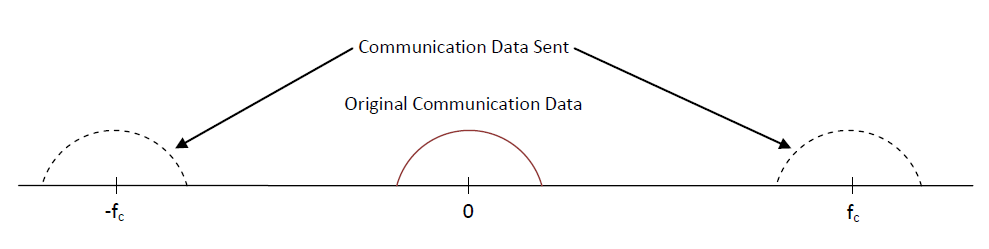
As can be seen from the figure above, in order to transmit <math>m(t)</math>, one must first process the data using a baseband processor (usually accomplished with software). As a result of this process the original data will be split into two signals, <math>x(t)</math> and <math>y(t)</math>, and shifted to a frequency <math>f_c</math> (and <math>-f_c</math> because of the notion of a complex conjugate). Note that the original wireless communication data that you want to send is in the form known as \textit{baseband}, which consists of frequencies near D.C. (or <math>f_c = 0</math>). When you actually send the communication data, however, you want to send it via much higher frequency (one which is inaudible to humans) and this creates a \textit{bandpass} signal. This concept is illustrated below: |
As can be seen from the figure above, in order to transmit <math>m(t)</math>, one must first process the data using a baseband processor (usually accomplished with software). As a result of this process the original data will be split into two signals, <math>x(t)</math> and <math>y(t)</math>, and shifted to a frequency <math>f_c</math> (and <math>-f_c</math> because of the notion of a complex conjugate). Note that the original wireless communication data that you want to send is in the form known as \textit{baseband}, which consists of frequencies near D.C. (or <math>f_c = 0</math>). When you actually send the communication data, however, you want to send it via much higher frequency (one which is inaudible to humans) and this creates a \textit{bandpass} signal. This concept is illustrated below: |
||
<br/> |
|||
<center>[[Image:HW2_BandpassBaseband.png]]</center> |
|||
<br/> |
|||
Why is the data split and shifted you ask? In the world of "Communication Systems" a signal to be transmitted can be written as |
Why is the data split and shifted you ask? In the world of "Communication Systems" a signal to be transmitted can be written as |
||
<br/> |
<br/> |
||
Revision as of 19:36, 8 April 2010
How It Works: Generalized Receiver
The process of transmitting data, , via a wireless signal, , is shown below:

As can be seen from the figure above, in order to transmit , one must first process the data using a baseband processor (usually accomplished with software). As a result of this process the original data will be split into two signals, and , and shifted to a frequency (and because of the notion of a complex conjugate). Note that the original wireless communication data that you want to send is in the form known as \textit{baseband}, which consists of frequencies near D.C. (or ). When you actually send the communication data, however, you want to send it via much higher frequency (one which is inaudible to humans) and this creates a \textit{bandpass} signal. This concept is illustrated below:
Why is the data split and shifted you ask? In the world of "Communication Systems" a signal to be transmitted can be written as
,
where is the signal to be sent and (Euler's identity) shifts the signal in the frequency domain by .
The above formula can then be rewritten to obtain the following relationship:
.
To visualize this process, observe the following figure:
This is why it is necessary to split into the the two signals . As you can also from the above relationship, in order to obtain the appropriate output signal one must multiply by a cosine function and by a negative sine function and then sum the results (this is illustrated in the figure above). In simplified terms and details, this is essentially how the data is transmitted.
The process of receiving data, , via a wireless signal, , is shown below:
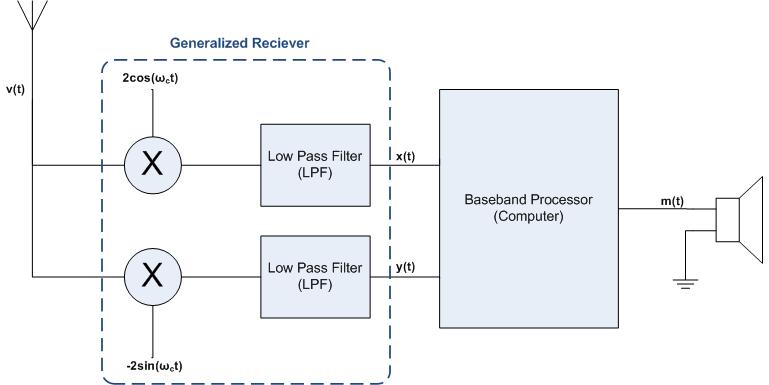
Receiving the original data from a wireless signal is very similar to that of transmitting the data (as described before). In short, the data that we are interested in () is embedded within the carrying signal ().







![{\displaystyle \displaystyle v(t)=Re[g(t)\cdot e^{j\omega _{c}t}]}](https://wikimedia.org/api/rest_v1/media/math/render/svg/84980ddc31ef70ba28686486029d15efd306809d)



![{\displaystyle \displaystyle v(t)=Re[g(t)\cdot e^{j\omega _{c}t}]=Re[(x(t)+jy(t))\cdot (\cos {\omega _{c}t}+j\sin {\omega _{c}t})=x(t)\cos {\omega _{c}t}-y(t)\sin {\omega _{c}t}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/23ed92e222b39e76a528ba824c1867ab5e3175d9)
