Fourier Example: Difference between revisions
Jump to navigation
Jump to search
Jorge.cruz (talk | contribs) |
No edit summary |
||
| (3 intermediate revisions by one other user not shown) | |||
| Line 8: | Line 8: | ||
== Solution == | == Solution == | ||
| Line 40: | Line 40: | ||
<math>f(x)=\frac{\pi}{2}+2(sin(x)+\frac{sin(3x)}{3}+\frac{sin(5x)}{5}+...)</math> | <math>f(x)=\frac{\pi}{2}+2(sin(x)+\frac{sin(3x)}{3}+\frac{sin(5x)}{5}+...)</math> | ||
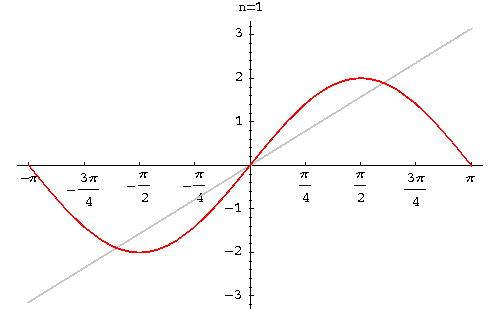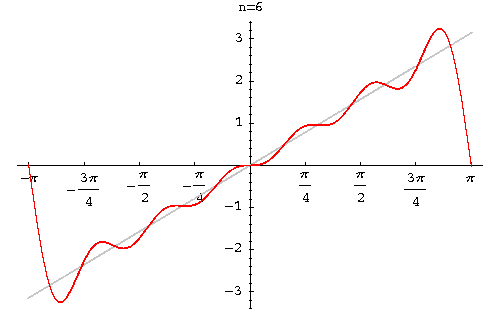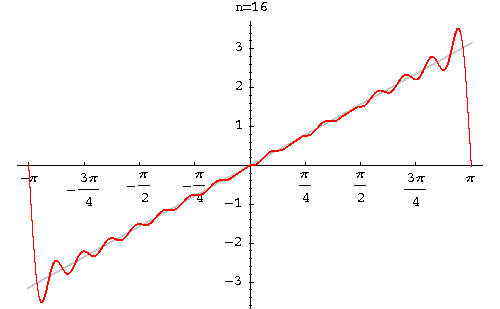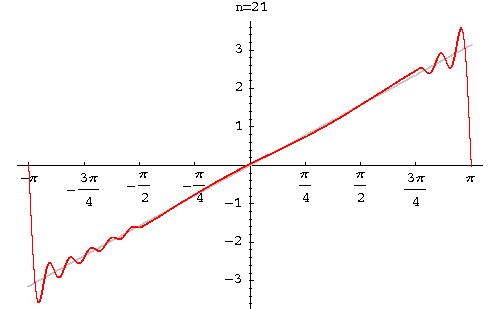
==Solution Graph== | |||
[[Image:Fourier.gif]] | |||
==***BONUS*** VIDEO! (For people completely lost on Fourier Series)== | |||
http://www.youtube.com/watch?v=nXEqrOt-nB8 | |||
==References:== | ==References:== | ||
[[Fourier Series: Basic Results]] | [[Fourier Series: Basic Results]] | ||
==Readers== | |||
[[Lau, Chris|Christopher Garrison Lau I]] | |||
Latest revision as of 15:26, 25 January 2010
Find the Fourier Series of the function:
Solution
Here we have
and
We obtain = 0 and
Therefore, the Fourier series of f(x) is
Solution Graph
***BONUS*** VIDEO! (For people completely lost on Fourier Series)
http://www.youtube.com/watch?v=nXEqrOt-nB8