Generalized Receiver Explanation: Difference between revisions
Jodi.Hodge (talk | contribs) No edit summary |
Jodi.Hodge (talk | contribs) No edit summary |
||
| (16 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
Human voice is heard near DC frequencies and is well suited to be packaged in a baseband signal. If a baseband signal is needed to be transmitted, the generalized transmitter will transform the baseband signal into a band pass signal, which has the benefit of requiring only half as much power. After the signal has been transmitted, the job of the generalized receiver is to transform the band pass signal, use for transmitting, back to the original base band signal. Circuit elements needed to create the generalized receiver are an oscillator, two low-pass filters, and two multipliers. The image below a block diagram from Dr. Frohne's Communication Systems class notes. |
|||
Generalized Receiver |
|||
<center>[[Image:Receiverblock.jpg]]</center> |
|||
Human voices are heard near DC frequencies and conviently the input signal from the antenna is a baseband signal. The job of the generalized receiver is to transform the base band signal into a base band signal that has an upper and lower part. The benefit of transforming the baseband signal to a band pass is that it requires only half the power to transmit it as band pass signals rather than a baseband signal.The circuit elements needed to create the receiver are an oscillator, two low-pass filters, and two multipliers. |
|||
---- |
|||
'''Experimental''' |
|||
Like what you will do in lab, to create a band pass signal will include the following stages. |
|||
''Stage one'' |
|||
'''''Oscillator''''' |
|||
Theoretically, Electronics class notes demonstrates how to create a band pass signal from a baseband signal. Lets called the baseband signal g(t). First, the baseband signal g(t) is multiplied by a function with both sine and cosine with frequency fc, <math>e^{j2 \pi f_{c}t}</math>.The results is the signal in the frequency domain shifted to the location of fc which now looks like G(f-fc). Next we take the real part of this signal, half the conjugate and the normal, is what we now call the band pass signal. |
|||
From your oscillator, manipulate the output take two signals; one that is twice the amplitude of the output and another that is twice the amplitude, inverted, and phase shifted by 90 degrees. These signals are <math>2cos(w_{c}t)\!</math> and <math>-2sin(w_{c}t))\!</math> in the block diagram. |
|||
''Stage two'' |
|||
'''''Mixers''''' |
|||
Lets call the band pass input into the receiver <math>v(t)\!</math>. Lets call the base band signal <math> g=x(t)+iy(t)\!</math>, where the two outputs of the receiver are its real and imaginary components. |
|||
Mixers work as multipliers. So the next step in building your general receiver is to use your mixers to perform multiplication of your input with your oscillator. Since we need two separate outputs, let’s have one mixer perform the multiplication of the band pass signal with cosine and the other mixer to perform the multiplication of the band pass signal with negative sine. |
|||
''Stage three'' |
|||
'''''Low pass filters''''' |
|||
The next stage in your receiver is to send the output of the multipliers into low pass filters (LPF). The LPF bandwidth is near to the frequency of the oscillator which will cut off all other frequencies we do not want. |
|||
---- |
|||
'''Theoretical''' |
|||
Theoretically, this how your convert a base band signal to a band pass signal. The band pass signal <math>v(t)\!</math> is the real part of the result of the complex baseband signal <math> g(t)\!</math> multiplied with <math>e^{j2 \pi f_{c}t}\!</math>. |
|||
| ⚫ | |||
| ⚫ | |||
<math>v(t)= x(t)cos(w_{c}t)-y(t)sin(w_{c}t)\!</math> |
|||
<center>[[Image:baseband.jpg]]</center> |
|||
The image shows how the base band signal then the band pass signal look in the frequency domain. |
|||
Using cool tricks with of sine and cosine and the low pass filter return<math>x(t)\!</math> and <math>y(t)\!</math> components of the original baseband signal. |
|||
This is the calculation that shows how the band pass signal, the oscillator, and the low pass filter are used. |
|||
<math>-LPF[v(t)2sin(w_{c}t)] \!</math> |
|||
The value of <math>v(t)\!</math> is substituted in |
|||
<math>-LPF[(x(t)cos(w_{c}t)-y(t)sin(w_{c}t)) *2sin(w_{c}t)]\!</math> |
|||
Multiplication distribution is performed |
|||
<math>-LPF[x(t)cos(w_{c}t)2sin(w_{c}t)-y(t)2sin^{2}(w_{c}t)]\!</math> |
|||
Trigonometry identities are used |
|||
<math>-LPF[x(t)sin(2w_{c}t)-y(t)(1-cos(w_{c}t)]\!</math> |
|||
The low pass filter is used to remove frequencies above wc, in this case all the terms with 2wc |
|||
<math>-LPF[x(t)sin(2w_{c}t)-y(t)(1-cos(2w_{c}t)]\!</math> |
|||
Experimentally, as you will be doing in lab, to create a band pass signal the process is as follows. First using your oscillator, you will need to create an original oscillating signal and one that is delayed by 90 degrees, this will be your sine and cosine like the one in the class notes. Next, you will use your two multipliers to perform the multiplication separately on the baseband signal. The next stage in your receiver is to pass the result of your multiplier into a low pass filter that has bandwidth of frequencies only close to the one of your oscillator to reduce the signal to noise ratio. Each signal from the low pass filter is output x(t) and quadrature y(t). In the soundcard that your receiver will be connected to will sum both signals together and would match the result we found theoretically. |
|||
The only signal that remains after the low pass filter is |
|||
| ⚫ | |||
<math> |
<math>y(t)\!</math> |
||
The same manipulation is done to get <math>x(t)\!</math> but rather than multiply the input by <math>-2sin(w_{c}t) \!</math> it is multiplied by <math>2cos(w_{c}t) \!</math> |
|||
| ⚫ | |||
Latest revision as of 17:32, 8 April 2010
Human voice is heard near DC frequencies and is well suited to be packaged in a baseband signal. If a baseband signal is needed to be transmitted, the generalized transmitter will transform the baseband signal into a band pass signal, which has the benefit of requiring only half as much power. After the signal has been transmitted, the job of the generalized receiver is to transform the band pass signal, use for transmitting, back to the original base band signal. Circuit elements needed to create the generalized receiver are an oscillator, two low-pass filters, and two multipliers. The image below a block diagram from Dr. Frohne's Communication Systems class notes.
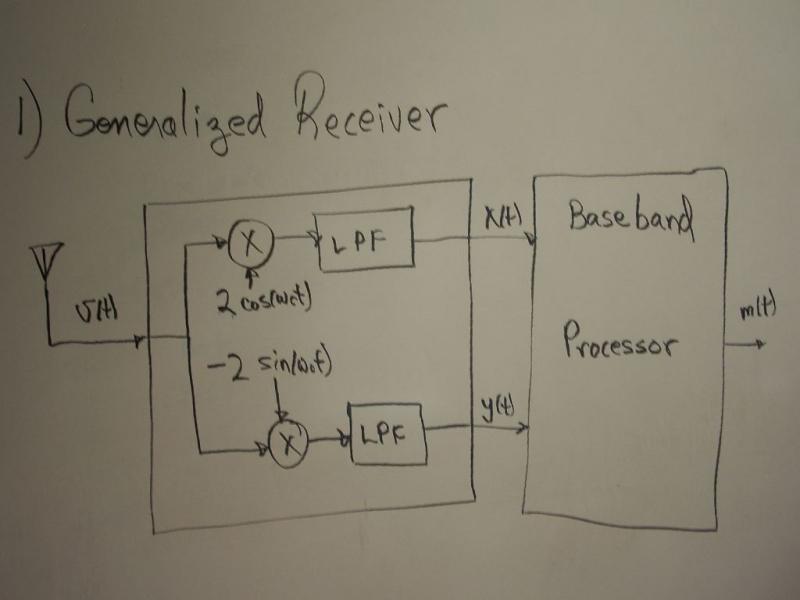
Experimental
Like what you will do in lab, to create a band pass signal will include the following stages.
Stage one
Oscillator
From your oscillator, manipulate the output take two signals; one that is twice the amplitude of the output and another that is twice the amplitude, inverted, and phase shifted by 90 degrees. These signals are and in the block diagram.
Stage two
Mixers
Lets call the band pass input into the receiver . Lets call the base band signal , where the two outputs of the receiver are its real and imaginary components.
Mixers work as multipliers. So the next step in building your general receiver is to use your mixers to perform multiplication of your input with your oscillator. Since we need two separate outputs, let’s have one mixer perform the multiplication of the band pass signal with cosine and the other mixer to perform the multiplication of the band pass signal with negative sine.
Stage three
Low pass filters
The next stage in your receiver is to send the output of the multipliers into low pass filters (LPF). The LPF bandwidth is near to the frequency of the oscillator which will cut off all other frequencies we do not want.
Theoretical
Theoretically, this how your convert a base band signal to a band pass signal. The band pass signal is the real part of the result of the complex baseband signal multiplied with .
![{\displaystyle v(t)=Re[g(t)e^{j2\pi f_{c}t}]\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/020e0d702a06860f216683185e5b5d68dea0b425)
![{\displaystyle v(t)=[(x(t)+jy(t))(cos(2\pi f_{c}t)+jsin(2\pi f_{c}t))]\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/1a12664f74ea9d420ace8e1d9f4fd28df88e117a)
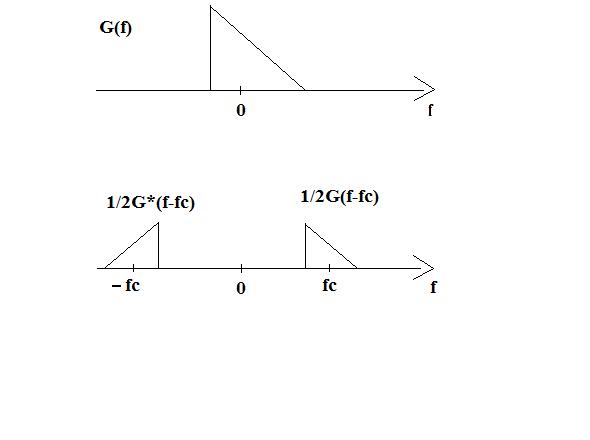
The image shows how the base band signal then the band pass signal look in the frequency domain.
Using cool tricks with of sine and cosine and the low pass filter return and components of the original baseband signal.
This is the calculation that shows how the band pass signal, the oscillator, and the low pass filter are used.
![{\displaystyle -LPF[v(t)2sin(w_{c}t)]\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/b638045c34be58cefb3944e1949bb8f083582046)
The value of is substituted in
![{\displaystyle -LPF[(x(t)cos(w_{c}t)-y(t)sin(w_{c}t))*2sin(w_{c}t)]\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/1cd454e890d62d1d8757832dc09da99b64d1c8a4)
Multiplication distribution is performed
![{\displaystyle -LPF[x(t)cos(w_{c}t)2sin(w_{c}t)-y(t)2sin^{2}(w_{c}t)]\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/7ea4ab95de0f663291baa1f0fcc9092f3addfbc1)
Trigonometry identities are used
![{\displaystyle -LPF[x(t)sin(2w_{c}t)-y(t)(1-cos(w_{c}t)]\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/9e9a6b0d4e4cb13101dc00881cc69ff158e1ad4c)
The low pass filter is used to remove frequencies above wc, in this case all the terms with 2wc
![{\displaystyle -LPF[x(t)sin(2w_{c}t)-y(t)(1-cos(2w_{c}t)]\!}](https://wikimedia.org/api/rest_v1/media/math/render/svg/21d29512ef0332a75700747ce4479526ce3f9d54)
The only signal that remains after the low pass filter is
The same manipulation is done to get but rather than multiply the input by it is multiplied by
