User:Caswto: Difference between revisions
Jump to navigation
Jump to search
| (10 intermediate revisions by the same user not shown) | |||
| Line 1: | Line 1: | ||
<center> | <center> | ||
[[Image:P1010004.JPG|thumb|9-27-2004]] <br> | |||
<br> | |||
Todd Caswell <br> | |||
509-374-2820 | |||
</center> | |||
---- | |||
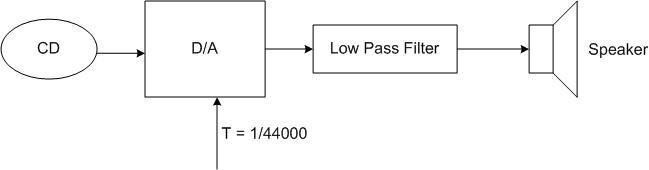
=== How a CD Player Works === | |||
[[Image:CDplayerdiagram.jpg|Description]] | |||
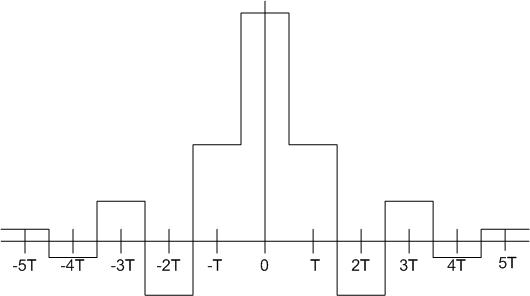
First, a digital signal <math>\ x(kt) </math> is read from the CD and then convolved with a pulse function <math>\ p(t) </math> in the D/A converter. The result in the time domain looks like this: | |||
<center> | <center> | ||
[[Image:DAOutput.jpg|Description]] | |||
<math> | |||
\hat x(t) = \sum_{k=-\infty}^\infty x(kT)p(t - kT) = p(t) *\sum_{k=-\infty}^\infty x(kT) \delta (t - kT) | |||
</math> | |||
</center> | </center> | ||
== | |||
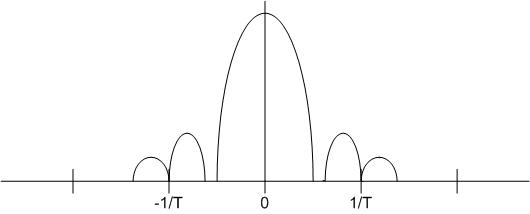
Let's look at this result in frequency space. Note that convolution in time means multiplication in frequency. | |||
<center> | |||
[[Image:DAfreqout.jpg|Description]] | |||
<math>\hat X(f) = 1/T \sum_{n=-\infty}^\infty X(f - n/T) \cdot P(f)</math> | |||
</center> | </center> | ||
where | |||
<center> | |||
<math>P(f) = \int_{-T/2}^{T/2} e^{j2\pi ft} \, dt = T sinc(fT) | |||
</math> | |||
</center> | |||
The low pass filter then knocks the high frequencies out of the signal coming from the D/A converter, which smoothes out the edges of the reproduced sine wave <math>\hat x(t)</math> in time. This output waveform then drives the speaker, thereby recreating the original sound stored on the CD. | |||
Contributing Authors: | |||
[[User:caswto|Todd Caswell]] | |||
[[User:goeari|Aric Goe]] | |||
Latest revision as of 11:09, 8 December 2004
Todd Caswell
509-374-2820
How a CD Player Works
First, a digital signal is read from the CD and then convolved with a pulse function in the D/A converter. The result in the time domain looks like this:
Let's look at this result in frequency space. Note that convolution in time means multiplication in frequency.
where
The low pass filter then knocks the high frequencies out of the signal coming from the D/A converter, which smoothes out the edges of the reproduced sine wave in time. This output waveform then drives the speaker, thereby recreating the original sound stored on the CD.
Contributing Authors: