An Ideal Transformer Example: Difference between revisions
Jump to navigation
Jump to search
No edit summary |
|||
| Line 8: | Line 8: | ||
<math>\ \omega=2\pi f</math>, so <math>\ \omega=120\pi</math> | <math>\ \omega=2\pi f</math>, so <math>\ \omega=120\pi</math> | ||
Therefore, <math>\ {e_{1}}(t)={ | Therefore, <math>\ {e_{1}}(t)=120\sqrt{2}\cos(120\pi t)V</math> | ||
Now the Thevenin equivalent impedance, <math>\ {Z_{th}}</math>, is found through the following steps: | Now the Thevenin equivalent impedance, <math>\ {Z_{th}}</math>, is found through the following steps: | ||
Revision as of 00:42, 19 January 2010
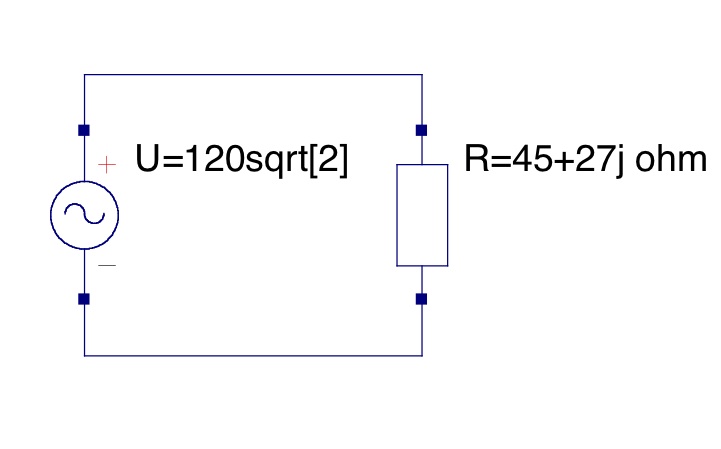
Consider a simple, transformer with two windings. Find the current provided by the voltage source.
- Winding 1 has a sinusoidal voltage of ° applied to it at a frequency of 60Hz.
- The combined load on winding 2 is
Solution
, so
Therefore,
Now the Thevenin equivalent impedance, , is found through the following steps:
Now, substituting:
Since ,
Since this is an ideal transformer, it can be modeled by this simple circuit:
Contributors
Read By
John Hawkins