Fourier Series: Explained!

A Brief Introduction

A Fourier series is a mathematical tool that takes a periodic function and turns it into a sum of simple oscillating functions (i.e. sines and cosines)<ref> Fourier Series</ref>. These series were discovered by Joseph Fourier to solve a heat equation in a metal plate.

How They Work

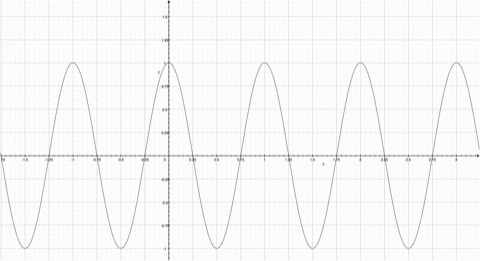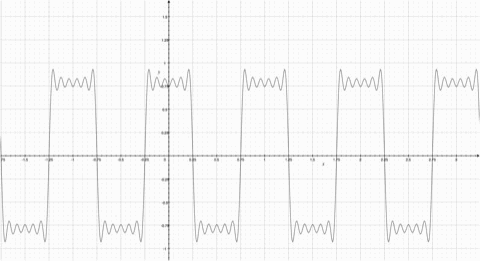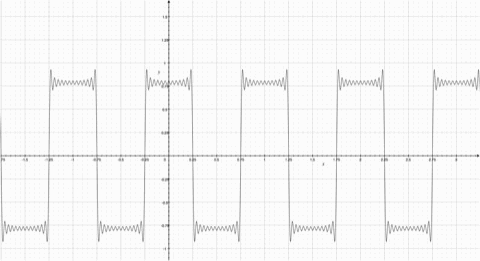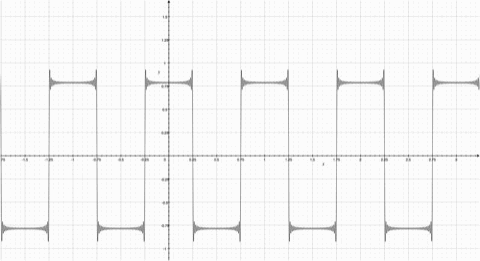
A Fourier Series represents a periodic function through a sum of sines or cosines. Each term in the summation has a frequency n. The first term has the same frequency as the periodic function, the second term has twice the frequency of the periodic function, and so on. The more functions added, the more the summation resembles the step function. Observe the animation; notice how the summation function resembles the original periodic function more as more functions are added.

Square Wave with similar periods to the cosine function

Fourier series animated to show increasing accuracy as evaluation bounds are increased.

References

<references/>

Helpful Links

A very helpful game

Contributors

• Christopher Garrison Lau I
• Michael Vier

Reviewed By

• Joshua Grant
• Vazquez, Brandon