Alex's Assignment

Alex's Fourier Project

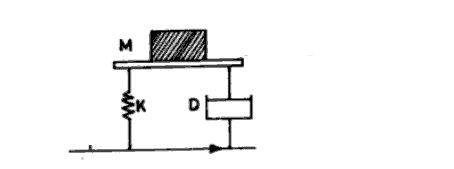
I chose to do my LNA project on a spring mass and dampener system such as what you would find on an automobile. As shown on the picture we have a mass (M), spring (K), and dampener (D).

General Equations

The first thing I need to do is write down the general equations for the Fourier Series:

${\begin{aligned}x(t)&=x(t+T)=a_{0}+\sum _{n=1}^{\infty }a_{n}\cos(n\omega _{0}t)+b_{n}\sin(n\omega _{0}t)\\\omega _{0}&=2\pi f_{0}\\a_{0}&={\frac {1}{T}}\int _{0}^{T}f(t)\,dt\\a_{n}&={\frac {2}{T}}\int _{0}^{T}f(t)\cos(n\omega _{0}t)\,dt\\b_{n}&={\frac {2}{T}}\int _{0}^{T}f(t)\sin(n\omega _{0}t)\,dt\\\end{aligned}}$

If odd function, $a_{n}=0$

If even function, $b_{n}=0$

Equations

Now that we have the basic equations for a Fourier Series we can begin to calculate for the spring dampener system. knowing that as the vehicle travels down a rough road it creates a displacement x we can equate the formula for the system. The forces apposing the displacement are:

The mass of the vehicle for one tire ${\frac {1}{4}}M$

The spring constant $Kx$

And the Dampening of the shock $Du$

Plugging in the forces into an Ordinary Differential Equation we get:

$Mu'+Du+Kx=0$