Alias
Aliasing
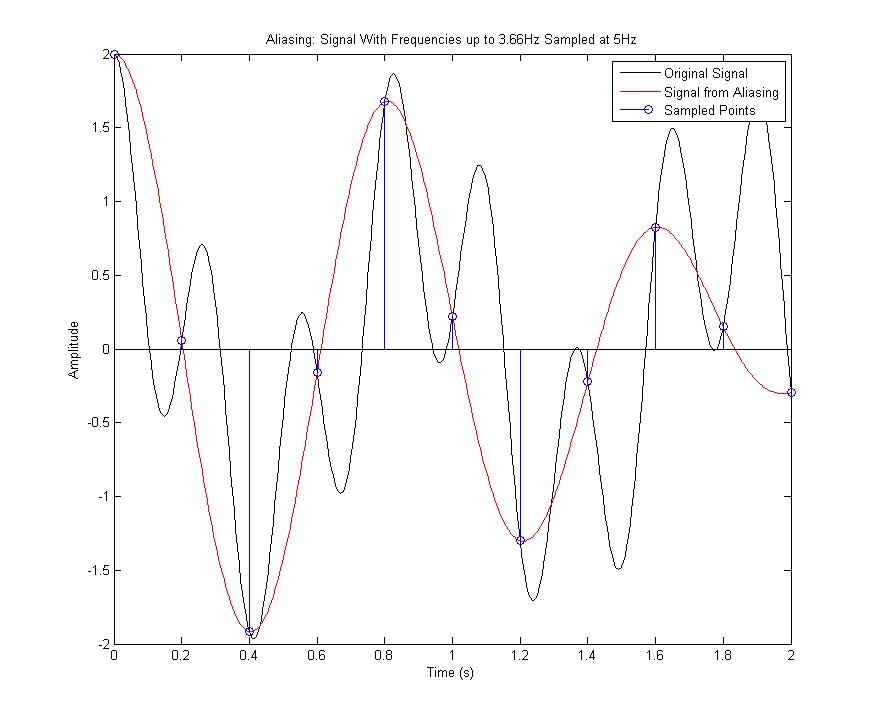
The Nyquist Theorem states that you must sample at a frequency which is twice that of the highest frequency components of the sampled signal in order to recover the sampled signal. If you sample at rate lower than this you get aliasing. Below is a figure which shows how aliasing occurs and the Matlab code for it.
clear all;
Ts = .2; %Sampling time (s)
ws = 2*pi/Ts; %Sampling frequency (rad/s)
t = [0:0.005:2]; %Time vector
w1 = 7; w2 = 23;
y = cos(w1*t) + cos(w2*t); %Original Signal
t1 = [0:Ts:2];
xs = cos(w1*t1) + cos(w2*t1); %Sampled points
w2s = w2 - ws;
x1 = cos(w1*t) + cos(w2s*t); %Signal from Aliasing
figure(1); %Plot
clf;
plot(t,y,'k',t,x1,'r');
hold on
stem(t1,xs);
hold off;
legend('Original Signal', 'Signal from Aliasing', 'Sampled Points');
xlabel('Time (s)');
ylabel('Amplitude');
title('Aliasing: Signal With Frequencies up to 3.66Hz Sampled at 5Hz');