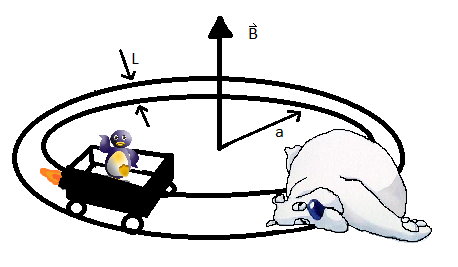
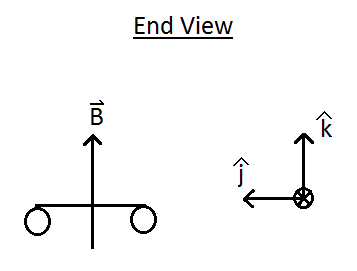
Example: Metal Cart

Problem

A DC generator is built using a metal cart with metallic wheels that travel around a set of perfectly conducting rails in a large circle. The rails are L m apart and there is a uniform magnetic ${\vec {B}}$ field normal to the plane. The cart has a penguin, a mass m, and is driven by a rocket engine having a constant thrust $F_{1}$. A wet polar bear, having stumbled out of a shack where he recently had a bad experience with a battery, lays dead across the tracks acting as if a resistor R is connected as a load. Find The current as a function of time. What is the current after the generator attains the steady-state condition?

Solution

For this Problem we will represent the large circle as a pair of straight parallel wires and the cart as a single wire. This is illustrated below in the top and end view figures

We have two forces, $F_{1}$ being the force from the rocket engine and $F_{2}$ being the force caused by the current in the conductor and the Magnetic Field. The resulting Force $F_{t}$ is simply the sum of $F_{1}$ and $F_{2}$

$F_{2}$ can be found using Ampere's Law

${\vec {F}}=\int \limits _{c}I~{\vec {d}}l\times {\vec {B}}~~~~~\Longrightarrow ~~~~~{\vec {F}}_{2}=\int \limits _{0}^{L}I~{\vec {d}}l\times {\vec {B}}~~~~~\Longrightarrow ~~~~~{\vec {F}}_{2}=-I(t)~B~L~~{\hat {i}}$

We can also say that $I(t)={\frac {-e_{m}(t)}{R}}$

And $~~{e_{m}(t)}=\int \limits _{o}^{L}({\vec {v}}\times {\vec {B}})~{\vec {d}}l~~~~~\Longrightarrow ~~~~~{e_{m}(t)}=-v~L~B$

$I(t)={\frac {v~L~B}{R}}~~~~~~~~~~~~~~~~~~~~~~{\vec {F}}_{2}=-I(t)~B~L~~{\hat {i}}~~~~~\Longrightarrow ~~~~~{\vec {F}}_{2}=-{\frac {v~L~B}{R}}~B~L~~{\hat {i}}~~~~~\Longrightarrow ~~~~~{\vec {F}}_{2}=-{\frac {v~L^{2}~B^{2}}{R}}~~{\hat {i}}$

${\dot {v}}={\frac {F_{1}}{m}}~{\hat {i}}~+{\frac {F_{2}}{m}}~{\hat {i}}~~~~~\Longrightarrow ~~~~~{\dot {v}}=~{\frac {F_{1}}{m}}~~{\hat {i}}~-{\frac {v~L^{2}~B^{2}}{Rm}}~~{\hat {i}}~~~~~\Longrightarrow ~~~~~{\dot {v}}~+~v\left({\frac {L^{2}~B^{2}}{R~m}}\right)~-~{\frac {F_{1}}{m}}~=~0$

Now we have a lovely differential equation to work with! To attempt to find the current we will take the Laplace transform.

${\mathcal {L}}\left\{{\dot {v}}~+~v\left({\frac {L^{2}~B^{2}}{R~m}}\right)-{\frac {F_{1}}{m}}\right\}~~~~~\Longrightarrow ~~~~~s~V(s)~+~{\frac {L^{2}B^{2}}{R~m}}V(s)~-~{\frac {F_{1}}{m~s}}$

Lets title and substitute in the variable $~~~~\psi ={\frac {L^{2}~B^{2}}{R~m}}~~$ to simplify things

$s~V(s)~+~\psi ~V(s)~-~{\frac {F_{1}}{m~s}}~=~0$

$V(s)~(s~+~\psi )~=~{\frac {F_{1}}{m~s}}~~~~~\Longrightarrow ~~~~~V(s)~=~{\frac {F_{1}}{m~s~(s~+~\psi )}}$

Using partial fraction expansion

${\frac {F_{1}}{m~s~(s~+~\psi )}}~~~~~~\Longrightarrow ~~~~~~{\frac {F_{1}/m\psi }{s}}~-~{\frac {F_{1}/m\psi }{s~+~\psi }}$

$V(s)={\frac {F_{1}/m\psi }{s}}~-~{\frac {F_{1}/m\psi }{s~+~\psi }}$

$V(t)={\mathcal {L}}\left\{{\frac {F_{1}/m\psi }{s}}~-~{\frac {F_{1}/m\psi }{s~+~\psi }}\right\}~~~~~\Longrightarrow ~~~~~V(t)={\frac {F_{0}}{m~\psi }}\left(u(t)~-~e^{-\psi ~t}~u(t)\right)~~~~~\Longrightarrow ~~~~~V(t)={\frac {F_{0}}{m~\psi }}\left(1~-~e^{-\psi ~t}\right)$

$V(t)={\frac {F_{0}}{m~{\frac {L^{2}~B^{2}}{R~m}}}}\left(1~-~e^{-{\frac {L^{2}~B^{2}}{R~m}}~t}\right)~~~~~\Longrightarrow ~~~~~V(t)={\frac {F_{0}~R}{L^{2}~B^{2}}}\left(1~-~e^{-{\frac {L^{2}~B^{2}}{R~m}}~t}\right)$

We know that $~~e_{m}(t)~=~V(t)LB$

So we can substitute in V(t) to get

$e_{m}(t)={\frac {F_{0}~R}{L~B}}\left(1~-~e^{-{\frac {L^{2}~B^{2}}{R~m}}~t}\right)$

And we know that $~~I(t)~=~{\frac {e_{m}(t)}{R}}$

$I(t)={\frac {{\frac {F_{0}~R}{L~B}}\left(1~-~e^{-{\frac {L^{2}~B^{2}}{R~m}}~t}\right)}{R}}~~~~~\Longrightarrow ~~~~~I(t)~=~{\frac {F_{0}}{L~B}}\left(1~-~e^{-{\frac {L^{2}~B^{2}}{R~m}}~t}\right)$

To find the steady-state current we simply look at the limit of I(t) as Failed to parse (unknown function "\infinity"): {\displaystyle T\rightarrow\infinity} . . . . .

In conclusion it can be seen that a penguin driven, polar bear killing generator would be a viable option for alternative energy in Canada.